Comparisons: Condorcet Methods 2

CHPV versus Condorcet Methods

Positional voting systems like CHPV do not generate Condorcet cycles or paradoxes. It is in the nature of the comparison between a pair of preferences that accounts for such anomalies. Let a voter award preference P_S and P_W to candidates A and B respectively; where P_S has a strong weighting and P_W has a weaker one. Hence, this voter increases the tally for A by +1 for the pairwise comparison between A and B. Relative to A, the tally for B has now declined by -1. As the difference in the rank positions of P_S and P_W is totally irrelevant, such positions are not explicitly stated. P_S and P_W may be the first and second preferences of a voter, the first and last ones or the penultimate and last preferences. For each pairwise comparison, each voter effectively awards either +1 or -1 to the differential tally.

Pairwise comparisons ignore the implied rank positions of the preferences and only focus on which is the higher one. Essentially, they therefore ignore the magnitude of the difference between the two preferences and only take note of the sign of this difference. Suppose a voter ranks A higher than B and B higher than C in a three-candidate election. This voter therefore adds +1 to the tally for A in comparison to B and +1 when comparing A to C too. This would normally imply an equality between B and C. Using differences in rank positions, if A is +1 higher than B and B is +1 higher than C then A is +2 higher than C in rank. Here the sums literally add up but for Condorcet methods they do not; as only the signs - and not the magnitudes - matter.

It is largely this disregard for the strength of voter preferences that accounts for the production of intransitive cycles and paradoxes in Condorcet method outcomes. Contradictory rankings are inevitable for some voter profiles. When faced with such anomalies, the various different types of Condorcet methods then try to recover from this lack of data by adopting additional ranking criteria to resolve the very situation that the basic Condorcet approach created.

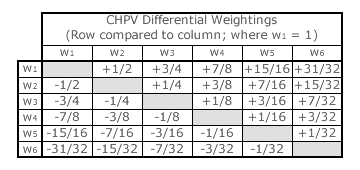
For CHPV and indeed all positional voting systems, both the sign and the magnitude of the difference between the two preference weightings matter. The table opposite provides the difference in weighting between any two preferences (for first to sixth preferences) for CHPV elections. Notice that each difference is unique. For example, a difference of +3/16 only results when a third preference (weighting = 1/4) is compared to a fifth preference (weighting = 1/16).

For plurality, the cells in the CHPV table would be replaced with +1 in the top row, with -1 in the left-hand column but with 0 elsewhere. Clearly, the differential weightings are not unique for plurality elections. For the Borda Count, the top row of cells would read +1, +2, +3, +4 and +5 points from left to right. More generally, where two preferences are separated by n rank positions, then the magnitude of the difference in their weightings is n points. The left-hand column of cells would hence read -1, -2, -3, -4 and -5 from top to bottom. Again, the differential weightings are not unique. For example, for any pair of adjacent preferences, the difference is +1 when comparing P_S to its neighbouring P_W in the Borda Count.

The major difficulty for Condorcet methods is that they ignore valuable information about the strength of voter preferences. This then often results in intransitive ranking cycles. Such paradoxes then have to be resolved by introducing extra complexity into the otherwise simple Condorcet algorithm. As the variant methods adopt different additional criteria, they have different properties. Consequently, they also often disagree as to the eventual winner when there is no outright Condorcet Winner. In contrast, any positional voting system such as CHPV employs an algorithm that always generates transitive outcome rankings so it never needs to modify or extend its basic algorithm. Also, CHPV differential weightings are always unique; unlike those for plurality or the Borda Count.

Proceed to next section > Comparisons: Alternative Vote (IRV)

Return to previous page > Comparisons: Condorcet Methods 1