Contents

Description of CHPV and GV

Introduction
Analogy
Weightings
Voting
Counting
Outcomes
Party-List
Summary

Evaluations of CHPV and GV

Ranked Ballot

Introduction (RB)
General Criteria
Majority Criteria
Clones & Teaming
Teaming Thresholds
Summary (RB)

Party-List

Introduction (PL)
Diagrams & Maps
CHPV Maps
Optimality
Party Cloning
Proportionality
Summary (PL)

Comparisons of CHPV with other voting systems

Single-Winner

Introduction (SW)
Plurality (FPTP)
Borda Count
Geometric Voting
Positional Voting
Condorcet Methods
AV (IRV)
Plur. Rule Methods
Summary (SW)

Multiple-Winner

Introduction (MW)
STV
Party-List
PL ~ Hare
PL ~ Droop
~ Maps Opt PC Pro
PL ~ D'Hondt
~ Maps Opt PC Pro
PL ~ Sainte-Laguë
~ Maps Opt PC Pro
Mixed Member Sys
Summary (MW)

Conclusions

Ranked Ballot CHPV
Party-List CHPV

General

Table of Contents

Map Construction

Table of Contents

Mathematical Proofs

Table of Contents
Notation & Formats

Valid XHTML 1.0 Strict

Valid CSS

Home About Description Evaluations(RB) Evaluations(PL) Comparisons(SW) Comparisons(MW) Conclusions General Maps Proofs
Home About Description Evaluations(RB) Evaluations(PL) Comparisons(SW) Comparisons(MW) Conclusions General Maps Proofs
Home > Proofs > Majority Criteria > EM3
Return to main text > Evaluations: Majority Criteria 3
Last Revision: 25 Apr 2021

Mathematical Proofs: Majority and Related Criteria

Proof EM3: Pairwise Majority Thresholds as a function of Common Ratio

Scenario: Candidate A has the proportion m of all first preferences cast (where 0 ≤ m ≤ 1). Candidate B has all the remaining first preferences; the proportion therefore being 1 - m. Candidate C has the second preference from every single voter; this whole proportion being 1. This scenario provides the consensus candidate C with the best opportunity to beat both the highly polarized candidates A and B despite C having no first preferences at all.

This worst case for A and B (and the best for C) is extended when those voters who awarded B their first preference truncate their ballot before ranking A so awarding A a zero weighting. Similarly, first preference A voters likewise truncate their ballot before ranking B. Effectively, supporters of one polarized candidate cannot bring themselves to award any positive value to the opposing polarized candidate.

Using standard notation and formats, the profile for this first election scenario is given below.

Alternatively, in an unlimited field of candidates (N → ∞), instead of truncation these first preference voters could award their lowest preference to the opposing polarized candidate as its weighting is vanishingly small (wN → 0).

Using standard notation and formats, the profile for this second election scenario is given below.

The standard GV weightings are used here to determine the tallies for candidates A, B and C. In both scenarios, each polarized candidate receives zero weightings from supporters of the other polarized candidate. The tallies for the remaining unnamed candidates are irrelevant as none of them can possibly beat C. For candidate A to avoid being beaten by B, the tally for A (TA) must be greater than or equal to the tally for B (TB). Similarly, the other two pairwise comparisons must be derived and then the overall rankings established. By evaluating the tallies, the required three pairwise majorities of first preferences (m) as a function of the common ratio (r) can be derived; as shown below.

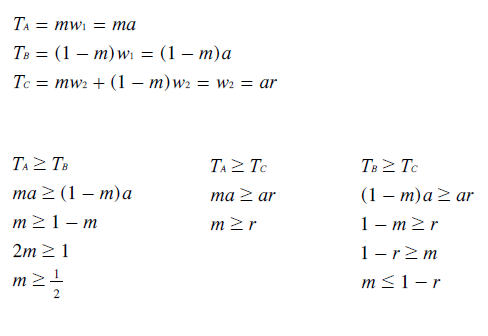
Proof EM3

The three thresholds are therefore m = 1/2, m = r and m = 1 - r. These are all straight line functions when plotted on a graph of m against r.


Return to main text > Evaluations: Majority Criteria 3

Refer to > Mathematical Proofs: Table of Contents